The weak interaction is said to be responsible for beta decay, muon decay, and some other decays. For example it’s said to be responsible for charged pion decay, but not for the more rapid neutral pion decay. That’s said to be caused by electromagnetism. However the electroweak interaction is said to be a unification of the weak interaction with electro-magnetism, and thus is said to cover all pion decays.
The beginnings of unification
As to when this unification began, it’s hard to say. Some might say it started with Hans Ørsted and electromagnetism in 1820. Some might say it started with Henri Becquerel and radioactivity in 1896. Some might say it started with Hermann Weyl and gauge invariance in 1929. But I think many would say it started with Enrico Fermi and his attempt at a theory of β rays in 1933. Hideki Yukawa then wrote a paper in 1935 on the interaction of elementary particles. In 1936 George Gamow and Edward Teller wrote a paper on the selection rules for the β-disintegration, and Alexandru Proca wrote a paper on the undulatory theory of positive and negative electrons. In 1938 Nick Kemmer wrote a paper that proposed a neutral current, Homi Bhabha wrote a paper on nuclear forces, heavy electrons, and the β-decay, and Oskar Klein wrote a paper on the theory of charged fields. But the war caused a hiatus, and there was much confusion to boot. See Viśvapriya Mukherji’s short history of the meson theory from 1935 to 1943 dating from 1971 for some history. Or see the articulation of theory: weak interactions by Allan Franklin and Slobodan Perovic dating from 2015.
Yang-Mills theory
Also see Tom Kibble’s history of electroweak symmetry breaking which again dates from 2015. He says “the first gauge theory beyond QED was the Yang-Mills theory [5], proposed in 1954, a gauge theory based on the isospin SU(2) symmetry”. He was referring to a paper by Chen-Ning Yang and Robert Mills called conservation of isotopic spin and isotopic gauge invariance:
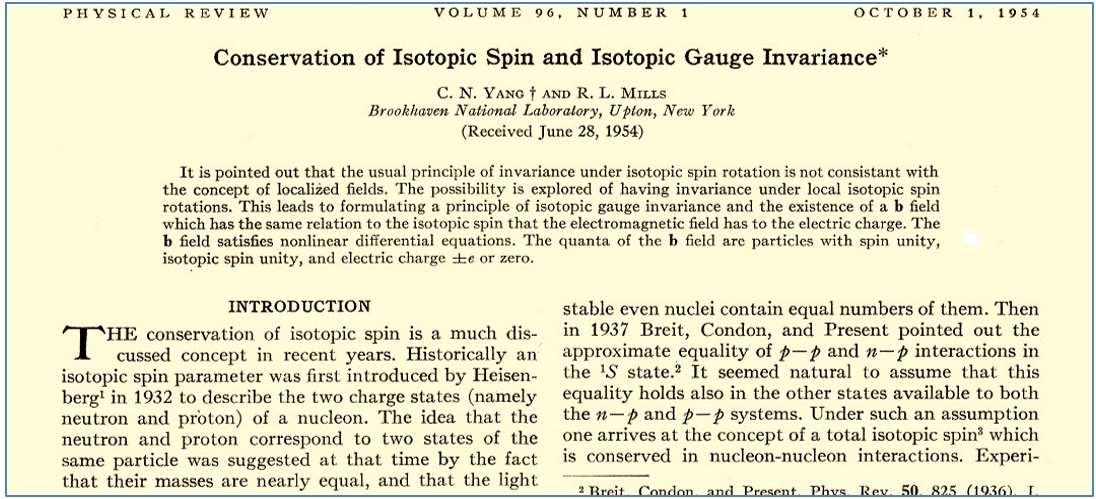
Yang and Mills talked about isotopic spin, which is the idea that the proton and the neutron are different spin states of the same particle. They also talked of gauge invariance, saying the relative orientation of the isotopic spin at two space-time points was a physically meaningless quantity. They defined isotopic gauge as an arbitrary way of choosing the orientation of isotopic spin axes, “in analogy with the electromagnetic gauge which represents an arbitrary way of choosing the complex phase factor of a charged field”. They talked about a b field, and about an isotopic spin current. They also talked about the quanta of this b field causing the proton and neutron to transform into each other. Wolfgang Pauli was famously critical, saying such gauge bosons had to be massless, otherwise it wasn’t a gauge theory. There’s also an issue wherein no such gauge bosons had been observed. Plus an issue in that the neutron and the proton are not different spin states of the same particle. Hence it’s perhaps not surprising that the Yang-Mills paper didn’t get much attention. There were no citations in 1954. There were two in 1955, four in 1956, and only one in 1957. See the 2015 physicsworld article From wrong to right Robert Crease. He says Yang–Mills theory was obviously wrong, but that it has since become fundamental to the Standard Model. However he say why. He says spontaneous symmetry breaking created a loophole around the Pauli snag, even though forces were supposed to arise from the conservation of symmetries. He also says Yang-Mills theories weren’t taken seriously until the 1970s, when they were shown to be renormalizable, not appreciating that renormalization is a kludge. Yang himself said the problem with the missing mass was resolved by the Standard Model, saying the Higgs field condensate “endows mass to elementary particles”. It seems he didn’t know about E=mc² or that the mass of a body is a measure of its energy-content.
Shaw and Utiyama miss out
Another 1954 contribution that didn’t get much attention was Ronald Shaw’s Invariance under general isotopic gauge transformations. That’s partly because it was part of his PhD thesis, which wasn’t completed until 1955. You can find it on page 110 of John Taylor’s 2001 book gauge theories in the twentieth century. Shaw said if a Lagrangian is invariant under translations and rotations in space-time, then momentum and angular momentum are conserved. He also said these two invariances “seem to exhaust the useful possibilities for transformations in space-time”. But that one can “postulate the invariance of the Lagrangian in a Euclidean space En of dimension n which has no relation to space-time, and useful results still follow”. As to how useful is debateable. Shaw also said invariance under rotation in an E2 space results in a divergenceless current vector and a conserved charge. He referred to a 1938 nuclear force paper by Nick Kemmer, a 1953 quantized fields paper by Julian Schwinger, and he talked of invariance under rotations in an E3 space, the “so-called isotopic spin space”. He also referred to the Salam-Polkinghorne scheme in which all the strong interactions are “assumed to be invariant under rotations in a space E4”. Christine Sutton gives Shaw a mention in the 1999 compilation it must be beautiful. She also refers to Ryoyo Utiyama and quotes Lochlainn O’Raifeartaigh saying Utiyama’s approach was the broadest and most comprehensive. She quotes Utiyama saying “I very much regret not submitting my paper to a Japanese journal in March 1954 when I had completed the work”. Utiyama’s eventual paper was called invariant theoretical interpretation of interaction:
It shares a common theme with the papers by Yang and Mills and by Shaw. It didn’t get much attention, which is perhaps not surprising. I say that because it displays no understanding of how electromagnetism works. Or what a photon is. Or an electron, or a proton, or a neutron, or a neutrino. It extends a mathematical principle with no physics foundation whatsoever.
Experiment to the rescue
However experiments were generating interest. The Cowan–Reines neutrino experiment in 1956 pinned down the neutrino. Clyde Cowan and Frederick Reines along with Kiko Harrison, Herald Kruse, and Austin McGuire wrote it up in their paper detection of the free neutrino: a confirmation:
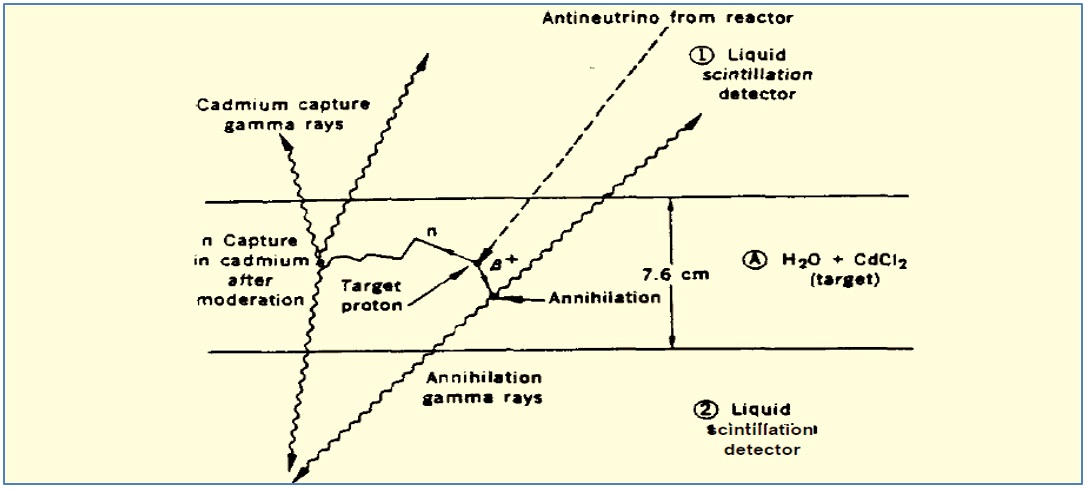Image from Frederick Reines’ 1995 Nobel lecture The Neutrino: From Poltergeist to Particle
In the same year Tsung-Dao Lee and Chen-Ning Yang wrote their parity-violation paper on the question of parity conservation in weak interactions. That was the recipe for the Wu experiment. Chien-Shiung Wu along with Ernest Abler, Raymond Hayward, Dale Hoppes, and Ralph Hudson wrote it up in their paper experimental test of parity conservation in beta decay. It was published in 1957 and led to Ennackal Sudarshan and Robert Marshak writing their V-A paper on the nature of the four-fermion interaction. That was circulated as a preprint in September 1957. A “related” paper was the theory of the Fermi interaction by Murray Gell-Mann and Richard Feynman. December 1957 then brought Nobel prizes for Yang and Lee. It also brought us the Goldhaber experiment, where Maurice Goldhaber, Lee Grodzins, and Andrew Sunyar demonstrated that neutrinos are left-handed. See their paper helicity of neutrinos. Things seemed to be coming together. As Tom Kibble said in his Scholarpedia article on the history of the Englert-Brout-Higgs-Guralnik-Hagen-Kibble mechanism, there was an “interesting possibility of a unified theory of weak and electromagnetic interactions, in which (W+, γ, W−) would form a triplet of gauge bosons”. However Kibble also said there were two severe problems. Since the weak interaction was short range, the W bosons had to have large masses, and it was generally believed that gauge bosons were massless. Then since the W bosons interacted via a parity-violating chiral current, they couldn’t reasonably be part of the same multiplet as the parity-conserving vector-current photon.
Schwinger was winging it
But the severe problems didn’t result in the re-appraisal of an idea that had started with Yukawa. Instead Julian Schwinger had a paper published in November 1957 on a theory of the fundamental interactions. He said “we shall attempt to describe the massive, strongly interacting particles by means of fields with the smallest spin appropriate to the statistics”. He then said this smallest spin was 0 for Bose-Einstein particles and ½ for Fermi-Dirac particles. That sounds odd given that there are no stable spin 0 particles, and a spin ½ particle has twice as much spin as a spin 1 particle. Then Schwinger said if the spin values are thus limited, “the origin of the diversity of known particles must be sought in internal degrees of freedom. We suppose that the various intrinsic degrees of freedom are dynamically exhibited by specific interactions, each with its characteristic symmetry properties”. That’s quite a leap, particularly since the only stable massive particles are the electron and the proton along with their antiparticles. Then he talked of transformations of the internal symmetry space and symmetry groups and sub-groups and group structures. He said “since the fundamental T=½ representation of the three-dimensional rotation group (familiar as isotopic spin) is first encountered within the framework of four-dimensional symmetries, it is natural to suppose that the latter is the underlying symmetry”. That’s another leap. Meanwhile there’s no attempt to focus on the simplest particles. Schwinger didn’t start with the photon or pair production. He didn’t explain the electron’s charge. Instead he talked of nucleonic charge and hypercharge, and leptonic charge and neutrinic charge. He cast his net wide and claimed that short-lived particles such as Ξ Σ Λ and Κ can be grouped into symmetrical families. Then on page 424 he said “the symmetry that exists between the heavy bosons and fermions in their isotopic space properties prompts us to ask: Is there also a family of bosons that realizes the T=1 representation of the three-dimensional rotation group?” He answered in the affirmative, making yet another leap. It was a leap of faith with no justification, like saying the knot zoo implies there’s a fishing-line zoo.
Knot zoo by Robert Scharein, see knotplot.com
But nevertheless Schwinger said “we are thus led to the concept of a spin one family of bosons”. He also spoke of massive charged Z particles and said the coupling with the Z field may be the underlying cause of “the whole group of physical processes that are characterized by a long time scale”. Schwinger was winging it. He’s like the detective who ignored the clues but who told you whodunnit on grounds of symmetry.
Glashow accepts the faith
One of Schwinger’s students was Sheldon Glashow, who gave some history in his Nobel lecture. He said this: “not only is electromagnetism mediated by photons, but it arises from the requirement of local gauge invariance”. Unfortunately it isn’t true. Electromagnetism is not mediated by photons. Virtual photons only exist in the mathematics of the model. Hydrogen atoms don’t twinkle, and magnets don’t shine. Moreover electrons and positrons don’t attract one another because of the requirement of local gauge invariance. They move the way they do because each is a dynamical “spinor” which alters the surrounding space akin to frame-dragging, thereby altering the motion of other spinors in that space. It’s akin to counter-rotating vortices attract, co-rotating vortices repel:
Glashow also said “this concept was generalized in 1954 to apply to non-Abelian local symmetry groups”. That’s true, but unfortunately it was generalized with no concept of the physical processes involved. Something else that’s true is that “a more far-reaching analogy might exist between electromagnetism and the other forces”. Of course it does. When you stop the current in the wires there’s still a force between the wires. What isn’t true is “they, too, might emerge from a gauge principle”. A gauge principle does not make the electron and positron circle towards one another as per ortho-positronium. Nor does it make the electron fall down. But Glashow accepted Schwinger’s faith, and said he was “led to the group SU(2) x U(1) by analogy with the approximate isospin-hypercharge group which characterizes strong interactions”. Glashow was perhaps also led by Sydney Bludman’s 1958 paper on the universal fermi interaction. That’s where Bludman predicted what’s now known as the Z boson, which was also predicted by José Leite Lopes in his 1958 paper on a model of the universal Fermi interaction. Glashow’s 1961 paper was partial symmetries of weak interactions. He said “at first sight there may be little or no similarity between electromagnetic effects and the phenomena associated with weak interactions. Yet certain remarkable parallels emerge”. That’s good. After all, the Wu experiment is reminiscent of the magnetic field around a current loop. What’s not so good is “the supposition that the weak interactions are mediated by unstable bosons”. There are no bosons flying back and forth between electrons and positrons, so why propose more? Particularly when Glashow himself said the mass of the charged intermediaries was the principal stumbling block. He said “without a theory of the origins of these masses, any study based upon the analogy between decay-intermediaries and photons may make use only of partial symmetries”. Glashow didn’t seem to know that the mass of body is a measure of its energy-content. Perhaps that’s why his paper didn’t have many citations in the early years. However the 1966 citation was by Peter Higgs, and the 1967 citation was by Steven Weinberg.
Weinberg’s electroweak unification paper
Weinberg’s 1967 paper was called a model of leptons, which it wasn’t. It is however only three pages long, and it is said to be the electroweak unification paper. Weinberg started by saying “leptons interact only with photons, and with the intermediate bosons that presumably mediate weak interactions”. Unfortunately it isn’t true. Electrons also interact with electrons, and with positrons and protons and neutrinos too. Don’t forget that Fermi’s interaction “posits four fermions directly interacting with one another”. In Weinberg’s model, they don’t. Weinberg talked of the neutral intermediate “meson” Zμ and said if it didn’t couple to hadrons then the best place to look for it effects is in electron-neutrino scattering. (He actually said electron-neutron scattering, but that was a typo). So, there’s an electron-neutrino interaction, but it isn’t because electrons interact with neutrinos. Instead it’s because of some massive particle that we can’t see. Weinberg also talked of electronic isospin T and hypercharge Y. (He actually said hyperchange, but that was another typo). He talked of a spin-zero doublet “whose vacuum expectation value will break T and Y and give the electron its mass”. Weinberg didn’t seem to know that the mass of body is a measure of its energy-content, or that the electron is a body. His Nobel lecture gives some insight to this. He talked about understanding symmetry, not the electron. He talked about renormalizability, as if it was a virtue rather than a kludge. He didn’t know about the hole in the heart of quantum electrodynamics, so he talked of isospin symmetry and said parity conservation was a sacred symmetry. But he also said “if symmetry principles are an expression of the simplicity of nature at its deepest level, then how can there be such a thing as an approximate symmetry?” Quite. Only he said he learned of a solid-state “broken symmetry” idea and fell in love with it. He then said “in at least one simple case the spontaneous breakdown of a continuous symmetry like isospin would necessarily entail the existence of a massless spin zero particle”. How does that work? When the proton and the neutron are said to be two spin states of the same particle, only they aren’t:
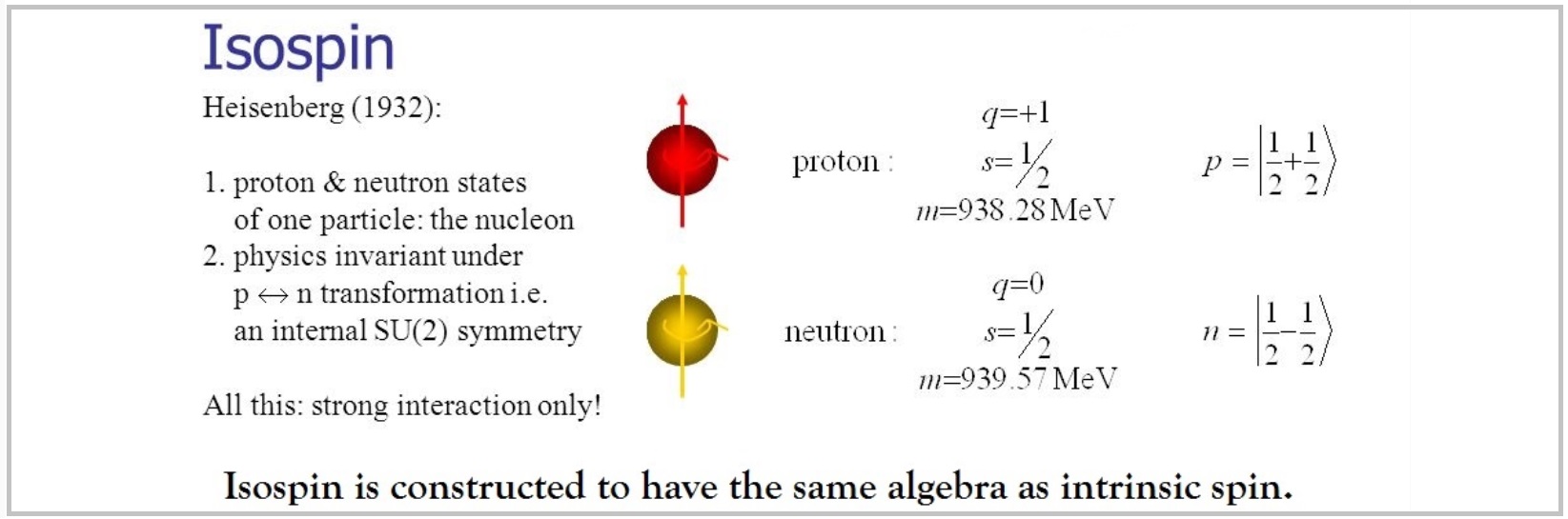Isospin image by William Coker
Especially when you break this so-called symmetry, you have a Goldstone boson which has never been observed? Weinberg said he had long discussions with Goldstone at Madison and then with Abdus Salam while he was his guest at Imperial College in 1961-62. He said “the three of us soon were able to show that Goldstone bosons must in fact occur whenever a symmetry like isospin or strangeness is spontaneously broken”. Even though they don’t occur. He said a solution was pointed out soon afterwards by Higgs and Kibble which meant the missing Goldstone bosons appear instead as helicity zero states of the vector particles, which thereby acquire a mass. He was talking about what’s now known as Weinberg’s toilet. He also said many thought what had been done was “to sweep the real problems under the rug”. Quite. In Weinberg’s model, the mass of the Z-boson is not a measure of its energy-content. Instead it gets its mass from “the spontaneous breaking of the symmetry”. Symmetry was touted as a great virtue, but when it didn’t work, broken symmetry was touted as a great virtue. Perhaps that’s why Weinberg’s paper didn’t have many citations in the early years. Amazingly he also said ”the naturalness of the whole theory is well demonstrated by the fact that much the same theory was independently developed [27] by Salam in 1968”. Even though they’d worked together.
Abdus Salam develops the theory independently
See the history of electroweak symmetry breaking by Tom Kibble, who joined Salam’s group at Imperial as a postdoc in 1959. Kibble said “the first of these symmetries was isospin”. He said it was proposed by Heisenberg, but doesn’t imply any physical relation to spin angular momentum. Instead it stems from “a mathematical similarity with the description of electron spin”. The idea is that the proton and the neutron can be regarded as two spin states of the same particle, and that there’s a symmetry under which one can rotate the proton into a neutron. On such is electroweak theory based. Kibble also referred to Nick Kemmer extending the symmetry to pions, and to a next step taken by Murray Gell-Mann and by Yuval Ne’eman, a student of Salam’s at Imperial. They each proposed a larger symmetry now known as the eightfold way. Kibble also said QED is a gauge theory, and that many people including Salam believed that viable theories of the other interactions should also be gauge theories. He referred to Yang and Mills, Shaw, Schwinger, Feynman and Gell-Mann, and Sudarshan and Marshak. He said Glashow “proposed an extended model with a larger symmetry group, SU(2) x (1), and a fourth gauge boson Z0”. He said in 1964 Salam and John Ward, apparently unaware of Glashow’s work, proposed a similar model in their paper electromagnetic and weak interactions. He also said a unified gauge theory of weak and electromagnetic interactions was proposed by Weinberg in 1967. And that “the same model was presented independently by Salam in lectures he gave at Imperial College in the autumn of 1967”. Kibble said Salam only published the following year, when he spoke of weak and electromagnetic interactions at a Nobel symposium in Sweden. That’s where Salam said “the only hope of achieving a renormalizable theory involving vector currents is that the mass of the meson should come through a spontaneous symmetry breaking”. Renormalization started as a fix for a failed theory, it ended up being touted as a virtue. Symmetry was a sacred principle, but symmetry breaking was needed as a fix:
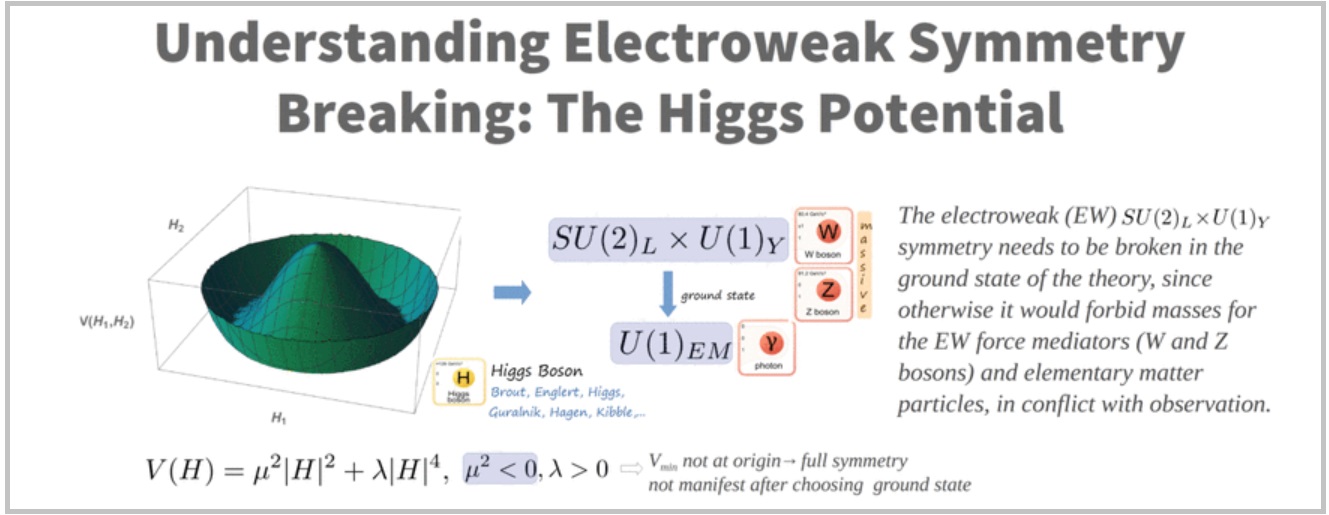Screenshot from electroweak symmetry breaking and the Higgs potential by Florian Goertz
Salam’s Nobel lecture is worth a read. It’s full of golden nuggets, such as “at that time CERN lived in a wooden hut just outside Geneva airport”. Or “the so-called weak nuclear force had to be mediated by some unknown spin-zero mesons if it was to be renormalizable”. There’s also this: “if so, the only eventual laboratory for particle physics will be the Early Universe, where we shall have to seek for the answers to the questions on the nature of charge”. I particularly liked “‘t Hooft’s work turned the Weinberg-Salam frog into an enchanted prince”. He was talking about Gerard ’t Hooft’s 1971 paper renormalization of massless Yang-Mills fields. And of course there’s also this: “I still remember Paul Matthews and I getting off the train at Aix-en-Provence for the 1973 European Conference and foolishly deciding to walk with our rather heavy luggage to the student hostel where we were billeted. A car drove from behind us, stopped, and the driver leaned out. This was Musset whom I did not know well personally then. He peered out of the window and said: “Are you Salam?” I said “Yes”. He said: “Get into the car. I have news for you. We have found neutral currents”.
We have found neutral currents
See the 2009 CERN courier article Gargamelle: the tale of a giant discovery by Don Cundy. He says the results in September 1973 came after two years work. And that Franz-Josef Hasert “realized that an event marked by a scanner as ‘muon plus gamma ray’ was in fact something more interesting: the clear signature of an electronic NC interaction written in the tracks of an electron knocked into motion by the punch of the unseen projectile”. The subsequent paper by Hasert et al was observation of neutrino like interactions without muon or electron in the Gargamelle neutrino experiment:
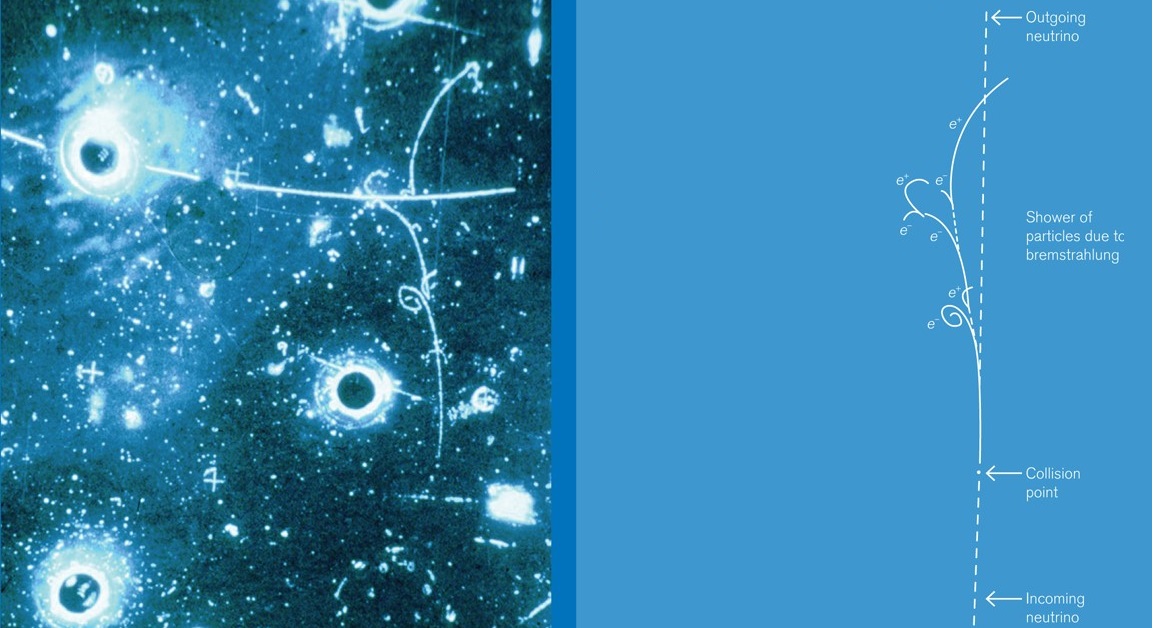CERN image from Symmetry magazine, see weak neutral current by Kurt Riesselmann
Also see the 2003 CERN courier article neutral currents by Don Perkins. He talks about a similar experiment at Fermilab by the HPWF (Harvard, Pennsylvania, Wisconsin, Fermilab) group, which in November 1973 came up with a neutral-current to charged-current ratio of 0.05. This was consistent with no neutral-current events. Perkins says the considerable uncertainties and errors in this experiment were eventually resolved and new values were given in April 1974 as NC:CC = 0.11 and 0.32. But “the damage had been done, and after this debacle many physicists were quite unconvinced of the reality of neutral currents”. This is perhaps understandable, because all they actually saw in the Gargamelle bubble chamber was electron tracks. Which could have been seen as proof of Fermi’s theory too. Or any theory that said neutrinos interact with electrons. Don’t forget that Compton scattering features neutral current in the guise of a photon, or that displacement current is a neutral current. Hence it’s perhaps understandable that CERN’s “discovery” was not acclaimed. Particularly since physicists were doubtless aware that CERN needed a discovery. See the discovery of the weak neutral currents by Dieter Haidt dating from 2004 where you can read that the discovery “brought CERN a leading role in the field… marked the experimental beginning of the Standard Model… triggered huge activity… led to the idea of building a proton-antiproton collider… justified building the Large Electron Positron collider”. It was the start of a long road for CERN, which once lived in a wooden hut near Geneva airport. But as per the 1998 CERN courier article twenty-five years of neutral currents, it started with raging controversy and nail-biting doubt. Plus the suspicion of cherry-picking. As Alexander Unzicker says on page 93 in his 2013 book, data analyzers selected just 100 events from 290,000 photographs. Unzicker points to the detailed account in Peter Galison’s How Experiments End, he quotes David Cline of HPWF describing the pressure he was under to conform, and he mentions Gary Taubes and his mocking reference to alternating neutral currents. No wonder there was no trip to Stockholm for six long years.
Cemented with a prize
The Nobel prize press release of October 1979 refers to the 1973 Gargamelle experiment, and to a 1978 experiment at the electron accelerator at SLAC in Stanford. That’s where “the scattering of high energy electrons on deuterium nuclei was studied and an effect due to a direct interplay between the electromagnetic and weak parts of the unified interaction could be observed”. The associated paper was parity non-conservation in inelastic electron scattering by Charles Prescott and nineteen other authors. They fired high-energy polarized electrons at a liquid deuterium target and measured the electrons deflected by 4° for various rotations of the polarizing calcite prism. An asymmetry of the electron flux was presented as proof of the Weinberg-Salam model of the weak interaction. A new era had begun. Particle physics had moved on from a particle tracks. Now the evidence was just a bump on a graph. But it was somehow enough for the Nobel committee and academy to decide what to do with the 1979 Nobel prize for physics. They awarded it to Glashow, Salam and Weinberg “for their contributions to the theory of the unified weak and electromagnetic interaction between elementary particles, including, inter alia, the prediction of the weak neutral current”.
Discovery of the W and Z bosons
The next major step was the discovery of the W and Z particles at CERN in 1983 which led to Nobel prizes for Carlo Rubbia and Simon van der Meer the following year. See the Wikipedia W and Z bosons article where you can read more, along with the 2005 CERN Courier article The W and Z particles: a personal recollection by Pierre Darriulat. But also see the January 2003 physicsworld article Carlo Rubbia and the discovery of the W and Z by Gary Taubes. It’s a shortened version of chapter 7 of his book Nobel Dreams: Power, Deceit, and the Ultimate Experiment. What comes across is the sheer Machiavellian intrigue and ruthlessness. Taubes tells how Rubbia would not be told that an event was not the detection of a W-boson. Rubbia leaked it to “establish his own impetuous version of a priority claim”. Then he took the event details to the USA later claiming that “we had a clear W signal in November”. Taubes tells how Rubbia showed the events to Salam, Weinberg, and Glashow, as well as the DoE. This is priceless: “The DoE’s Bernie Hildebrand asked Rubbia to sign the computer-generated image of the event and then mounted it on his wall. Later, Hildebrand heard second or third hand that Burt Richter of the Stanford Linear Accelerator Center had seen the event and he did not believe it was a W. Hildebrand added a note to the picture that read ‘Carlo, this is not a W – Burt’”. Taubes goes on to tell how Rubbia sold the “discovery” of the W boson to a willing audience at CERN in January 1983. He got a standing ovation, even though what had actually been observed was a high-energy electron:
Images from Carlo Rubbia and the discovery of the W and Z by Gary Taubes
Taubes also tells how Rubia persuaded the rival UA2 collaboration to think twice before publishing, so giving Rubbia time to beat them into print. Then in May 1983 Rubbia did it all over again with the Z boson. The event was referred to as the Z-zero, and it was not gold-plated, because whilst one electron was perfect the other was not. But that didn’t stop CERN director-general Herwig Schopper announcing that the Z boson had been discovered. Schopper wanted Nobel prizes for CERN physicists. He “wanted to secure the future of the large electron positron (LEP) collider at CERN. Although he had persuaded the governments of CERN’s member states to pay for the machine, he would still have to fight every year to keep the money coming in against a grim economic background in Europe. LEP was a $500m project and Schopper needed international prestige for the project. The Nobel prize would provide that prestige”. When a Church needs a miracle, a Church gets a miracle.
And here we are
And here we are. The W and Z bosons are said to be mediators of the electroweak interaction, which is said to be settled science. It is said that the W-boson is not responsible for neutral pion decay, which takes circa 8.4 x 10ˉ17 seconds. However it is said to be responsible for charged pion decay which takes circa 2.6 x 10ˉ8 seconds, as well as muon decay which takes circa 2.2 x 10ˉ6 seconds, and neutron decay which takes circa 8.8 x 102 seconds. But what causes W-boson decay, which takes circa 3 x 10ˉ25 seconds? And what causes Z-boson decay, which again takes circa 3 x 10ˉ25 seconds? Silence. And how on Earth can an 80 MeV particle be responsible for muon decay? Is it somehow magicked up, spontaneously, like worms from mud?
Public domain image by Thymo, see Wikipedia
Some would say yes. See for example the video particle physics 5 by Dr Physics. At 2 minutes 17 seconds he says the Wˉ boson is 80 times heavier than the neutron from which is was formed, and that the question is how can something that weighs 1 GeV produce something that weighs 80 GeV? His answer is that the requisite energy can be borrowed from the vacuum provided that a) you pay it back and b) you don’t keep it for very long. Dr Physics also says the Wˉ only lasts 10ˉ25 seconds and can barely cross a part of a proton, and that’s why the weak nuclear force is very short range. You don’t need to know that spin is real, or that virtual particles only exist in the mathematics of the model, or about Homi Bhabha’s catch-22 where he tried to explain beta decay with an intermediary particle that decays spontaneously, to know that this is lies to children. That this is foundation-free handwaving horseshіt. And yet the Standard Model is built of this. What a disaster it is.